How To Find Median Using Formula

How to find median using excel, how to find median using cumulative frequency, how to find median using spss, how to find median using pdf, how to find median using mean, how to find median, how to find median class, how to find median from histogram, how to find slope, how to screenshot on windows, how to draw,

When it comes to statistics, there are a plethora of concepts to understand and apply in order to get a clear picture of data sets. One such concept is mean deviation about median, which we will be delving into today. Understanding mean deviation about median requires a good grasp on basic statistics concepts such as mean, median, and deviation. But fear not, we will be explaining everything step-by-step so that you can fully understand what mean deviation about median is and how to calculate it.

Basic Statistics Concepts

Before we dive into mean deviation about median, let's first take a moment to understand some basic statistics concepts that will be important to our discussion.

  • Mean - The mean, also known as the average, is the sum of all values in a data set divided by the number of values.
  • Median - The median is the middle value of a data set when the values are arranged in order of magnitude.
  • Deviation - Deviation is a measure of how spread out the values in a data set are. It is calculated by subtracting the mean from each value and then squaring the result. The sum of all squared differences is then divided by the number of values, and the square root is taken to get the average deviation.

Mean Deviation about Median

Now that we have a good grasp on basic statistics concepts, let's move on to mean deviation about median. Mean deviation about median is a measure of variation that shows how much variation there is in a data set relative to the median. In other words, it measures the average distance between each value in a data set and the median.

To calculate mean deviation about median, we first arrange the values in the data set in order of magnitude. We then find the median of the data set. Next, we subtract the median from each value and take the absolute value. We then calculate the mean of these absolute differences. The result is the mean deviation about median.

Let's take a look at an example to illustrate this concept:

Example

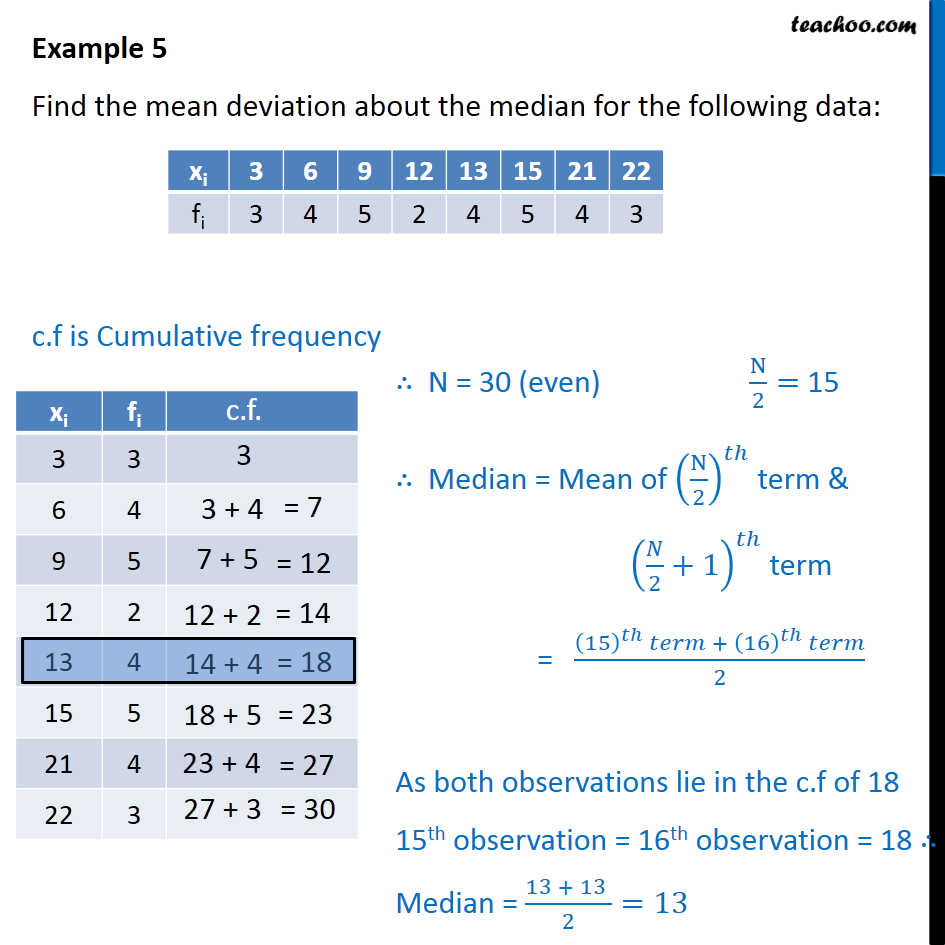
Here we have a data set with the following values: 2, 4, 6, 8, 10. To calculate mean deviation about median, we first arrange the values in order of magnitude:

2, 4, 6, 8, 10

The median value is 6, since it is the middle value when the values are arranged in order of magnitude. Next, we find the absolute value of the difference between each value and the median:

  • 2 - 6 = 4
  • 4 - 6 = 2
  • 6 - 6 = 0
  • 8 - 6 = 2
  • 10 - 6 = 4

We then calculate the mean of these absolute differences:

(4 + 2 + 0 + 2 + 4) / 5 = 2.4

So the mean deviation about median for this data set is 2.4.

Conclusion

Mean deviation about median is an important concept to understand in statistics. It is a measure of variation that shows how much variation there is in a data set relative to the median. Calculating mean deviation about median requires a good grasp on basic statistics concepts such as mean, median, and deviation. By following the steps we outlined in this post, you should now be able to easily calculate mean deviation about median for any data set.


Also read:

.